Q1(a) The sign of work done by a force on a body is important to understand. State carefully if the following quantities are positive or negative:

work done by a man in lifting a bucket out of a well by means of a rope tied to the bucket.

Answer:

In this case, the direction of force and displacement are the same, this work done is positive.

Q1(b) The sign of work done by a force on a body is important to understand. State carefully if the following quantities are positive or negative:
(b) work done by gravitational force in the above case

Answer:

In this case, the direction of displacement is upward and the direction of force is downward. Thus work done is negative in nature.

Q1 (c) The sign of work done by a force on a body is important to understand. State carefully if the following quantities are positive or negative: work done by friction on a body sliding down an inclined plane,

Answer:

We know that friction acts in the direction opposite to the direction of motion. Hence work done by the frictional force is negative.

Q 1 (d) The sign of work done by a force on a body is important to understand. State carefully if the following quantities are positive or negative: work done by an applied force on a body moving on a rough horizontal plane with uniform velocity

Answer:

In this case, the applied force supports the motion of the object (balances frictional force). Thus work done by the force is positive.

Q1 (e) The sign of work done by a force on a body is important to understand. State carefully if the following quantities are positive or negative: work done by the resistive force of air on a vibrating pendulum in bringing it to rest.

Answer:

Work done in this case is negative as the direction of force and motion are not identical.

Q2 (a) A body of mass 2 kg initially at rest moves under the action of an applied horizontal force of 7 N on a table with coefficient of kinetic friction = 0.1. Compute the work done by the applied force in 10 s,

Answer:

Using Newton's law we can write :

The frictional force is given by :

Its direction will be opposite of the direction of the motion. Thus acceleration produced will be negative.

Thus the net acceleration is = 3.5 - 0.98 = 2.52 m/s ² .

The total distance travelled is given by :

or

Hence the work done by applied force is given by :

Q2 (b) A body of mass 2 kg initially at rest moves under the action of an applied horizontal force of 7 N on a table with coefficient of kinetic friction = 0.1. Compute the work done by friction in 10 s,

Answer:

The work done by frictional force will be negative as the force opposes the motion.

Q2 (c) A body of mass 2 kg initially at rest moves under the action of an applied horizontal force of 7 N on a table with coefficient of kinetic friction = 0.1. Compute the work done by the net force on the body in 10 s,

Answer:

The net work done will be the sum of work done by applied force and work done by frictional force.

Q2 (d) A body of mass 2 kg initially at rest moves under the action of an applied horizontal force of 7 N on a table with coefficient of kinetic friction = 0.1. Compute the change in kinetic energy of the body in 10 s

Answer:

It is given that initial velocity is zero. The final velocity can be calculated by the equation of motion :

or

or

Thus change is kinetic energy is :

or

or

Q3 Given in Fig. 6.11 are examples of some potential energy functions in one dimension. The total energy of the particle is indicated by a cross on the ordinate axis. In each case, specify the regions, if any, in which the particle cannot be found for the given energy. Also, indicate the minimum total energy the particle must have in each case. Think of simple physical contexts for which these potential energy shapes are relevant.

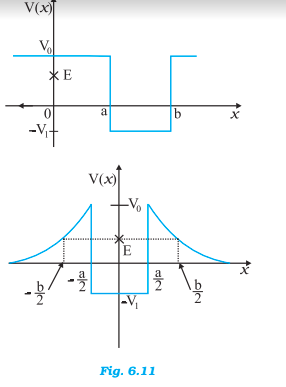
Answer:

Total energy = kinetic energy (KE) + potential energy(PE)

KE > 0 since m and v ² is positive. If KE <0 particles cannot be find. If PE>TE, then KE<0 (now in all graph check for this condition)

In case 1 kinetic energy is negative for x<a. So at x<a particle cannot be found.

In case 2 for x<a and for x> b kinetic energy is negative. So the particle cannot be found in these regions.

In the third case , the minimum potential energy is when . At this position, the potential energy is negative (- V ₁ ).

The kinetic energy in this case is given by :

And the minimum energy of particle is - V ₁ .

In the fourth case , the particle will not exist in the states which will have potential energy greater than the total energy.

Thus particle will not exist in and .

The minimum energy of particle will be - V ₁ as it is the minimum potential energy.

Q4 The potential energy function for a particle executing linear simple harmonic motion is given by , where k is the force constant of the oscillator. For the graph of V(x) versus x is shown in Fig. 6.12. Show that a particle of total energy 1 J moving under this potential must ‘turn back’ when it
reaches

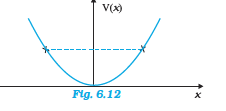
Answer:

The total energy of the particle is given by :

or

At the extreme position, the velocity of the object is zero thus its kinetic energy at that point is zero.

or

or

or

Hence the extreme position are .

Q5 (a) Answer the following :
The casing of a rocket in flight burns up due to friction. At whose expense is the heat energy required for burning obtained? The rocket or the atmosphere?

Answer:

The total energy is given by :

or

The burning of casing results in a reduction in the mass of the rocket. This leads to a lowering in the total energy.

Thus heat required for burning is obtained from the expenses of the rocket .

Q5 (b) Comets move around the sun in highly elliptical orbits. The gravitational force on the comet due to the sun is not normal to the comet’s velocity in general. Yet the work done by the gravitational force over every complete orbit of the comet is zero. Why?

Answer:

This is because the gravitational force is a conservative force. And we know that the work done by a conservative force in a closed path is always zero. That's why the work done by the gravitational force is zero in a complete orbit revolution.

Q5 (c) An artificial satellite orbiting the earth in very thin atmosphere loses its energy gradually due to dissipation against atmospheric resistance, however small. Why then does its speed increase progressively as it comes closer and closer to the earth?

Answer:

The total energy of artificial satellite remains constant. Thus when it approaches towards the earth the distance between them decreases. This results in a decrease in the potential energy of the satellite. By energy conservation, the kinetic energy of satellite increases and so does the velocity.

Q5 (d) In Fig. 6.13(i) the man walks 2 m carrying a mass of 15 kg on his hands. In Fig. 6.13(ii), he walks the same distance pulling the rope behind him. The rope goes over a pulley, and a mass of 15 kg hangs at its other end. In which case is the work done greater?

Answer:

In the first case,

Work done is :

or

or

or

In the second case :

or

or

Thus work done in the second case is greater than the first case.

Q6 (a) When a conservative force does positive work on a body, the potential energy of the body increases/decreases/remains unaltered.

Answer:

It is given that work done by the conservative force is positive, thus the force acts in the direction of the motion. This results in a decrease in distance between the bodies. Thus it's potential energy decreases .

Q6 (b) Work done by a body against friction always results in a loss of its kinetic/potential energy.

Answer:

Work done by the body against friction results in a decrease in the velocity of the body. Thus the kinetic energy of the body decreases.

Q6 (c) The rate of change of total momentum of a many-particle system is proportional to the external force/sum of the internal forces on the system.

Answer:

The internal force cannot produce a change in the total momentum as no external force is acting. Thus the change in total momentum is proportional to the external forces acting on the body.

Q6(d) In an inelastic collision of two bodies, the quantities which do not change after
the collision are the total kinetic energy/total linear momentum/total energy of
the system of two bodies.

Answer:

The conservation of total linear momentum doesn't depend upon the fact whether it is an elastic collision or an inelastic collision.

Q7(a) State if each of the following statements is true or false. Give reasons for your answer.

In an elastic collision of two bodies, the momentum and energy of each body is conserved.

Answer:

False: - The linear momentum and energy will be conserved if both are considered in a system. But for individual bodies, this conservation of momentum and energy doesn't hold. This is because the impact during the collision may transfer energy/momentum of one ball to the other ball.

Q7 (b) State if each of the following statements is true or false. Give reasons for your answer. (b) Total energy of a system is always conserved, no matter what internal and external forces on the body are present.

Answer:

False: - Internal forces will not change the energy of the system but external forces can change the total energy by changing their magnitude or direction.

Q7 (c) State if each of the following statements is true or false. Give reasons for your answer. Work done in the motion of a body over a closed loop is zero for every force in nature.

Answer:

False:- This is true only for conservative forces e.g. gravitational force. For e.g in case of frictional force (non-conservative force), the work done in a closed-loop cannot be zero as energy is wasted throughout.

Q7 (d) State if each of the following statements is true or false. Give reasons for your answer. In an inelastic collision, the final kinetic energy is always less than the initial kinetic energy of the system.

Answer:

True but not always:- In the case of inelastic collisions, few amounts of energy is converted into other forms of energy such as sound or in deformation. Thus final kinetic energy is always less as compared to initial kinetic energy. But in case of the explosion of a bomb final kinetic energy is greater than the initial kinetic energy

Q8 (a) Answer carefully, with reasons: In an elastic collision of two billiard balls, is the total kinetic energy conserved
during the short time of collision of the balls (i.e. when they are in contact)?

Answer:

No, because at the time of the collision, the kinetic energy is converted to the potential energy. Thus total kinetic energy is not constant at the collision.

Q8 (b) Is the total linear momentum conserved during the short time of an elastic collision of two balls?

Answer:

Yes, in case of elastic collision the total linear momentum of the system remains conserved as no external force is acting on the system of balls.

Q8 (c) What are the answers to (a) and (b) for an inelastic collision?

Answer:

The total kinetic energy of the system cannot be conserved in case of inelastic collision as there is loss of energy in the form of deformation. But the total linear momentum of the system remains constant even in the case of inelastic collision as no external force is acting.

Q8 (d) If the potential energy of two billiard balls depends only on the separation distance between their centres, is the collision elastic or inelastic? (Note, we are talking here of potential energy corresponding to the force during collision, not gravitational
potential energy).

Answer:

Since the potential energy of the system depends upon the separation between the bodies thus the forces acting on the body are conservative in nature. We know that conservative forces produce elastic collisions.

Q 9) A body is initially at rest. It undergoes one-dimensional motion with constant acceleration. The power delivered to it at time t is proportional to

Answer:

It is given that acceleration is constant thus force will also be constant (by Newton's law of motion F = ma).

Also,

or

Thus

Now, the work done by the force is given by :

Hence power is directly proportional to the time.

Q10 A body is moving unidirectionally under the influence of a source of constant power. Its displacement in time t is proportional to

Answer:

We know that the power is given by :

or

or

It is given that power is constant, thus :

or

By integrating both sides, we get

Also, we can write :

or

By integrating we get the relation :

Q11 A body constrained to move along the z-axis of a coordinate system is subject to a constant force F given by
where are unit vectors along the x-, y- and z-axis of the system respectively. What is the work done by this force in moving the body a distance of 4 m along the z-axis?

Answer:

Force is given to be :

And the displacement is :

Thus the work done is given by :

or

or

Q12 An electron and a proton are detected in a cosmic ray experiment, the first with kinetic energy 10 keV, and the second with 100 keV. Which is faster, the electron or the proton? Obtain the ratio of their speeds. (electron mass , proton mass
)

Answer:

The kinetic energy of the electron is given by :

or

Thus velocity is obtained as :

or

Similarly, we can find the velocity of the proton :

Thus velocity is obtained as :

or

Thus the ratio of their velocities is :

Q13 A rain drop of radius 2 mm falls from a height of 500 m above the ground. It falls with decreasing acceleration (due to viscous resistance of the air) until at half its original height, it attains its maximum (terminal) speed, and moves with uniform speed thereafter. What is the work done by the gravitational force on the drop in the first and second half of its journey? What is the work done by the resistive force in the entire journey if its speed on reaching the ground is ?

Answer:

The volume of the drop is :

Thus the mass of raindrop is :

or

Thus the work done is given by :

or

or

or

Now the total energy at the peak point is :

or

or

And the energy at the ground is :

or

or

Thus work done by the resistive force is :

Q14 A molecule in a gas container hits a horizontal wall with speed and angle 30 ^o with the normal, and rebounds with the same speed. Is momentum conserved in the collision? Is the collision elastic or inelastic?

Answer:

The momentum is conserved in the collision as no external force is acting on the system. In the given case the rebound velocity is the same as the initial velocity thus the kinetic energy of the molecule initially and finally are same. Hence this is an elastic collision.

Q15 A pump on the ground floor of a building can pump up water to fill a tank of volume 30 m ³ in 15 min. If the tank is 40 m above the ground, and the efficiency of the pump is 30%, how much electric power is consumed by the pump?

Answer:

Mass of the water is :

or

Thus the output power is given by :

or

or

or

Also, we are given that efficiency is 30 per cent.

Thus the input power is :

or

Q16 Two identical ball bearings in contact with each other and resting on a frictionless table are hit head-on by another ball bearing of the same mass moving initially with a speed V. If the collision is elastic, which of the following (Fig. 6.14) is a possible result after collision?

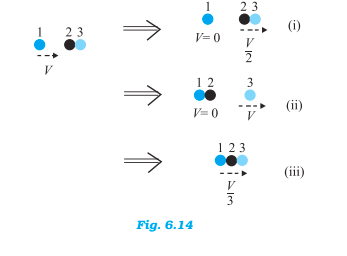
Answer:

The initial kinetic energy of the system is given by :

or

Case (i):- The final kinetic energy is :

Thus the kinetic energy is not conserved in this case.

Case (ii):- The final kinetic energy is :

Thus kinetic energy is conserved in this case.

Case (iii):- The final kinetic energy is:-

or

Thus the kinetic energy is not conserved in this case.

Q17 The bob A of a pendulum released from 30o to the vertical hits another bob B of the same mass at rest
on a table as shown in Fig. 6.15. How high does the bob A rise after the collision? Neglect the size of
the bobs and assume the collision to be elastic.

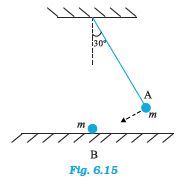
Answer:

This is an elastic collision thus the transfer of momentum will take place. It is given that bob B is at rest and bob A has some velocity. So in momentum transfer, bob B will gain the velocity in the left direction whereas bob A will come to rest (complete momentum transfer takes place).

Hence bob A will not rise.

Q18 The bob of a pendulum is released from a horizontal position. If the length of the pendulum is 1.5 m, what is the speed with which the bob arrives at the lowermost point, given that it dissipated 5% of its initial energy against air resistance?

Answer:

Consider the extreme position (horizintal) :-

The kinetic energy at this position is zero as velocity is zero.

Thus total energy is given by :

Now consider the mean position (lowermost point) :

Here the potential energy of bob is zero.

Whereas kinetic energy is :

Further, it is given that 5 per cent of energy is dissipated due to air resistance while coming down.

Thus energy equation becomes (conservation of energy):-

or

Q19 A trolley of mass 300 kg carrying a sandbag of 25 kg is moving uniformly with a speed of 27 km/h on a frictionless track. After a while, sand starts leaking out of a hole on the floor of the trolley at the rate of . What is the speed of the trolley after the entire sandbag is empty?

Answer:

Since the sand is falling in the trolley thus the force generated on the system (trolley and sandbag) is an internal force. There is no external force thus momentum of the system doesn't change. Hence speed remains the same i.e., 27 Km/hr.

Q20 A body of mass 0.5 kg travels in a straight line with velocity where . What is the work done by the net force during its displacement from x = 0 to x = 2 m ?

Answer:

The relation between work done and the kinetic energy is given by :

Using the relation we can write :

Initial velocity = 0 (at x = 0 )

And the final velocity = (at x = 2).

Thus work done is :

or

or

Q21 (a) The blades of a windmill sweep out a circle of area A. If the wind flows at a velocity v perpendicular to the circle, what is the mass of the air passing through it in time t?

Answer:

The volume of wind = here is the swept circle and is the velocity.

Thus the mass of the wind is : - , is the density of the air.

Hence mass of wind flowing through windmill in time t is _.

Q21 (b) The blades of a windmill sweep out a circle of area A. What is the kinetic energy of the air?

Answer:

The kinetic energy is given by :

or

or

Thus the kinetic energy of wind is J.

Q21 (c) The blades of a windmill sweep out a circle of area A. Assume that the windmill converts 25% of the wind’s energy into electrical energy, and that and the density of air is What is the electrical power produced?

Answer:

It is given that 25 per cent of wind energy is converted into electrical energy.

Thus electric energy produced is :

or

Now the electric power is given by :

or

or

or

Q22 (a) A person trying to lose weight (dieter) lifts a 10 kg mass, one thousand times, to a height of 0.5 m each time. Assume that the potential energy lost each time she lowers the mass is dissipated. How much work does she do against the gravitational force?

Answer:

The work done against the gravitational force is given by :

= Number of times the weight is lifted work done in 1 time.

or

or

Q22 (b) A person trying to lose weight (dieter) lifts a 10 kg mass, one thousand times, to a height of 0.5 m each time. Assume that the potential energy lost each time she lowers the mass is dissipated. Fat supplies of energy per kilogram which is converted to mechanical energy with a 20% efficiency rate. How much fat will the dieter use up?

Answer:

Efficiency is given to be 20 per cent.

Thus energy supplied by the person :

Thus the amount of fat lost is :

or

Q23 (a) A family uses 8 kW of power. Direct solar energy is incident on the horizontal surface at an average rate of 200 W per square meter. If 20% of this energy can be converted to useful electrical energy, how large an area is needed to supply 8 kW?

Answer:

It is given that the efficiency of energy conversion is 20 per cent.

According to question, we can write (equating power used by family) :

(Here A is the area required.)

or

or

Thus required area is 200 m ² .

Q23 (b) A family uses 8 kW of power. Compare this area to that of the roof of a typical house.

Answer:

A typical has dimensions of .

The area of the roof of the house is .

This is nearly equal to the area required for the production of the given amount of electricity.

NCERT Solutions for Class 11 Physics Chapter 6 Work Energy and Power Additional Exercise

Q24 A bullet of mass 0.012 kg and horizontal speed strikes a block of wood of mass 0.4 kg and instantly comes to rest with respect to the block. The block is suspended from the ceiling by means of thin wires. Calculate the height to which the block rises. Also, estimate the amount of heat produced in the block.

Answer:

We are given :

Mass of the bullet m: 0.012 Kg

Mass of the block M: 0.4 Kg

The initial velocity of the bullet u: 70 m/s

The initial velocity of the block : 0

The final velocity of the system (bullet + block): v

For finding the final speed of system we will apply the law of conservation of momentum :

or

Now for the system, we will apply the law of conservation of energy :

The potential energy at the highest point = Kinetic energy at the lowest point

or

or

or

Hence heat produced is :

or

or

Q25 Two inclined frictionless tracks, one gradual and the other steep meet at A from where two stones are allowed to slide down from rest, one on each track (Fig. 6.16). Will the stones reach the bottom at the same time? Will they reach there with the same speed? Explain. Given and h = 10 m, what are the speeds and times taken by the two stones?

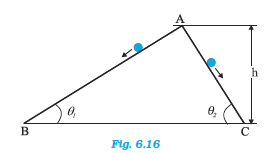
Answer:

The FBD of the track is shown in the figure below :

Using the law of conservation of energy we have :

or

Hence both stones will reach the bottom with the same speed.

For stone 1 we can write :

or

For stone 2 we have :

Also, using the equation of motion,

or

It is given that

or

Thus

Hence, the stone travelling on the steep plane will reach before.

For finding speed and time we can use conservation of energy.

or

or

or

And the time is given by :

and

Q26 A 1 kg block situated on a rough incline is connected to a spring of spring constant as shown in Fig. 6.17. The block is released from rest with the spring in the unstretched position. The block moves 10 cm down the incline before coming to rest. Find the coefficient of friction between the block and the incline. Assume that the spring has a negligible mass and the pulley is frictionless.

Answer:

Displacement (x) of the block is given as : = 0.1 m.

Using equilibrium conditions we can write :

and ( is the frictional force).

We can write work done in terms of potential energy as :

or

or .

Thus the coefficient of friction is 0.125.

Q27 A bolt of mass 0.3 kg falls from the ceiling of an elevator moving down with an uniform speed of . It hits the floor of the elevator (length of the elevator = 3 m) and does not rebound. What is the heat produced by the impact? Would your answer be different if the elevator were stationary?

Answer:

In this case, the heat produced is the loss in the potential energy.

Thus,

heat produced = mg h

or

or

The heat produced (when the lift is stationary) will remain the same as the relative velocity of the bolt with respect lift still remains zero.

Q28 A trolley of mass 200 kg moves with a uniform speed of 36 km/h on a frictionless track. A child of mass 20 kg runs on the trolley from one end to the other (10 m away) with a speed of relative to the trolley in a direction opposite to its motion, and jumps out of the trolley. What is the final speed of the trolley ? How much has the trolley moved from the time the child begins to run ?

Answer:

The initial momentum of the system (boy + trolley) is given as :

Now assume v ' is the final velocity of the trolley with respect to the ground.

Then the final momentum will be :

Conserving momentum :

or

The time taken by the boy is :

Hence the distance moved by the trolley is :

Q29 Which of the following potential energy curves in Fig. 6.18 cannot possibly describe the elastic collision of two billiard balls? Here r is the distance between centres of the balls.

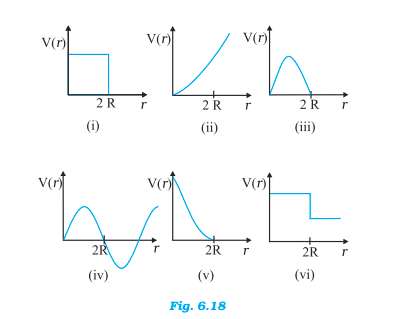Answer:

The potential energy of the system depends inversely on the separation between the balls. Thus the potential energy will decrease as the balls will come closer and will become zero as they touch each other.

Thus elastic collision is best described only by the graph (v).

Q30 Consider the decay of a free neutron at rest: Show that the two-body decay of this type must necessarily give an electron of fixed energy and, therefore, cannot account for the observed continuous energy distribution in the of a neutron or a nucleus (Fig. 6.19).

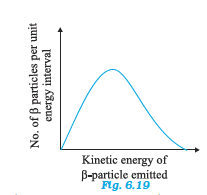
[Note: The simple result of this exercise was one among the several arguments advanced by W.
Pauli to predict the existence of a third particle in the decay products of This
particle is known as the neutrino. We now know that it is a particle of intrinsic spin ½ (like
e—, p or n), but is neutral, and either massless or having an extremely small mass
(compared to the mass of an electron) and which interacts very weakly with matter. The
correct decay process of the neutron is : ]

Answer:

By Einstein’s mass-energy relation we can write :

Here and C are constant thus two-body decay is unable to explain (or account for) the continuous energy distribution in the β -decay of a neutron.